Pythagorean intonation.

A tuning of the scale in which all 5ths and 4ths are pure (untempered). Pythagorean tuning provides intonations of several types of scale. A series of five 5ths and 4ths includes the pitch classes of the most familiar kind of pentatonic scale; ascending from F

 the series would comprise the five chromatic notes of the keyboard. A series of seven 5ths ascending from F yields a diatonic scale comprising the naturals on the keyboard; the 3rds and 6ths in this scale, however, differ from their justly intoned equivalents by a syntonic comma, and therefore do not meet medieval and Renaissance criteria of consonance implied by such terms as ‘perfection’ and ‘unity’. When used as harmonic intervals these Pythagorean 3rds and 6ths are likely to be characterized, on an organ Diapason stop for example, by rather prominent Beats; middle C–E or C–A beat more than 16 times per second at modern concert pitch. A series of 12 Pythagorean 5ths provides a fully chromatic scale that is bound to include, however, one sour Wolf 5th, smaller than pure by a Pythagorean comma.

Traditional Pythagorean theory is a matter of string-length ratios (on the Monochord) between multiples of 2 and 3, but of no larger prime numbers. (For ancient, medieval and Renaissance theorists the larger number in each ratio normally represented the greater string length and thus the lower pitch.) The ratio for the octave is 2:1, and for the 5th 3:2. To find the ratio for the sum of two intervals their ratios are multiplied; to find the ratio for the intervallic difference, the ratios are divided. The ratio for the 4th is 4:3 (octave – 5th, hence 2:1 ÷ 3:2); for the whole tone, 9:8 (5th – 4th, hence 3:2 ÷ 4:3); for the major 6th, 27:16 (5th + whole tone, hence 3:2 × 9:8); for the minor 3rd, 32:27 (octave – major 6th, hence 2:1 ÷ 27:16, or 4th – whole tone, hence 4:3 ÷ 9:8); for the ‘ditone’ or major 3rd, 81:64 (whole tone + whole tone, hence 9:8 × 9:8); for the ‘limma’ or diatonic minor 2nd, 256:243 (4th – major 3rd, hence 4:3 ÷ 81:64); for the somewhat larger ‘apotomē’, 2187:2048 (whole tone – limma, hence 9:8 ÷ 256:243) and so on.

Among regular tuning systems Pythagorean intonation has the largest major 2nds and 3rds and smallest minor 2nds and 3rds. Melodically the large major 2nds are handsome and the incisiveness of the small minor 2nds is of potential expressive value. Hence Pythagorean intonation is well suited not only to parallel organum but also to late Gothic polyphonic compositions in which the role of harmonic major 6ths is somewhat analogous to that of dominant 7th chords in later triadic music, while the use of double leading-note cadences as in ex.1 places a premium on the incisive melodic quality of the small semitones. Medieval theorists who discussed intervallic ratios nearly always did so in terms of Pythagorean intonation.

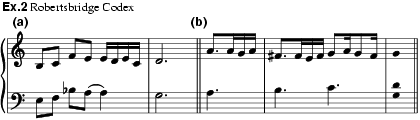
Of particular significance for the development of harmony in Western music was the use of Pythagorean intonation on early Renaissance keyboard instruments. The repertory of the Robertsbridge Codex (GB-Lbl Add.28550) shows that a fully chromatic keyboard was in use by about 1340, and passages like ex.2 suggest that at that time the tuner would set the chromatic scale by adding pure 5ths at both ends of the chain of 5ths forming the chromatic scale, leaving the wolf 5th perhaps betwen G

 and E. But by the time of the early 15th-century liturgical keyboard repertory of the Faenza Codex (I-FZc 117), the five chromatic notes seem to have been tuned to make a chain of pure 5ths among themselves, leaving the wolf 5th between B and F. It happens that what might be called a Pythagorean diminished 4th (e.g. the interval between the first and last members of the following chain of pure 5ths or 4ths: B–E–A–D–G–C–F–B–E) actually forms a much more nearly pure major 3rd than does the diatonic Pythagorean 3rd itself. Hence all the triangles in fig.1 would represent virtually pure triads, or at least particularly sonorous ones, if all the 5ths shown in the spiral were tuned pure (with special care to make none larger than pure). In this disposition of Pythagorean intonation, which may be referred to conveniently as the F × B disposition, each of the five chromatic degrees falls within the lower half of the diatonic whole tone. This disposition was prescribed or referred to by numerous 15th-century theorists, including Prosdocimus de Beldemandis, Ugolino of Orvieto, Johannes Keck, Henri Arnaut de Zwolle, a certain ‘librum Baudeceti’ cited by Arnaut, Johannes Gallicus, John Hothby (referring specifically to the organ), Nicolò Burzio, Franchinus Gaffurius (in his Theorica musica of 1492), and Heinrich Schreiber; its traces are also found in the 1472 portrayal of a clavichord in the ducal palace at Urbino (see Clavichord, fig.3). 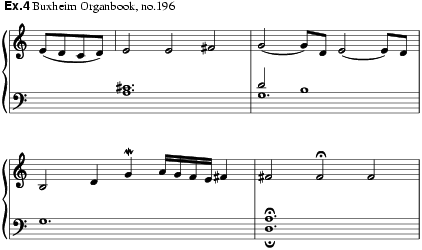There is corroborating musical evidence, mostly from the first half of the century: exx.3a and b, the conclusions of two organ verses, are typical of the evidence to be found in the liturgical Faenza Codex repertory. Exx.4 and 5 are from two settings in the Buxheim Organbook (D-Mbs 3725) of Binchois’ Adieu ma tres belle; other pieces in the Buxheim repertory that exploit the especially resonant triads of the F × B disposition of Pythagorean intonation include nos.19, 30–31, 126–8, 141, 153–5, 180 and 242. Most of these are keyboard settings of songs initially composed in the first half of the 15th century. (Most of the original keyboard compositions in the Buxheim Organbook, including all the fundamenta, seem to require some form of regular mean-tone temperament for their proper effect; see Temperaments, §§2 and 3.) The cadence in ex.6, the opening of a Buxheim transcription of Du Fay’s Mille bon jours, would not have, in the F × B disposition, the high leading note cited above as a virtue of Pythagorean intonation in Gothic cadences; nonetheless the pure or nearly pure intonation of the quasi-dominant triad sounds very good in this and other such Dorian contexts. The F × B disposition of Pythagorean intonation, in addition to having perhaps abetted the development of tonality by promoting what might anachronistically be called half-cadences in the Dorian mode, evidently whetted that Renaissance appetite for sonorous triads which only mean-tone temperaments could fully satisfy on keyboard instruments.

Although Gaffurius and other late 15th-century opponents of the theoretical innovations advanced by Ramis de Pareia cited the Pythagorean F

 × B scheme as the proper alternative to Ramos’s new monochord, Gaffurius in 1500 changed to an ostensibly Pythagorean monochord of 14 pitch classes forming a chain of 5ths ascending from A

 to D

. In 1496 Gaffurius had acknowledged, however, that organists tempered their 5ths when tuning the instrument. Pietro Aaron and G.M. Lanfranco, the first Italian writers to give tuning instructions for mean-tone temperaments (in 1523 and 1532 respectively), were, like Gaffurius, unabashed upholders of Pythagorean theory in contradiction to their own descriptions of practice. This dichotomy between ‘speculative’ and practical accounts of musical intervals became so pronounced during the 16th century that when Simon Stevin, the Dutch mathematician and engineer, worked out his precise calculations for equal temperament around 1600, he was familiar with Pythagorean theory but only dimly aware of the existence of mean-tone temperaments, which were subsequently described to him by a musician friend, Abraham Verheyen.

BIBLIOGRAPHY

Boethius: De institutione musica (Venice, 1491–2); Eng. trans. C.M. Bowen in C.V. Palisca, ed.: Boethius: Fundamentals of Music (New Haven, CT,1989)

C.E. Naumann: Über die verschiedenen Bestimmungen der Tonverhältnisse und die Bedeutung des pythagoreischen oder reinen Quinten-Systems für unsere heutige Musik (Leipzig, 1858)

J.M. Barbour: ‘The Persistence of the Pythagorean Tuning System’, Scripta mathematica, i (1932–3), 286

H. Stephani: Zur Psychologie des musikalischen Hörens (Regensburg, 1956)

M. Kolinski: ‘A New Equi-Distant 12-Tone Temperament’, JAMS, xii (1959), 210–14

C.V. Palisca: Girolamo Mei: Letters on Ancient and Modern Music (Rome, 1960)

F.R. Levin: The Harmonics of Nicomachus and the Pythagorean Tradition (Philadelphia, 1975)

B. Münxelhaus: Pythagoras musicus (Bonn, 1976)

E.C. Pepe: ‘Pythagorean Tuning and its Implications for the Music of the Middle Ages’, The Courant, i/2 (1983), 3–16

For further bibliography see Temperaments and Just intonation.

MARK LINDLEY