(Ger. Umkehrung).
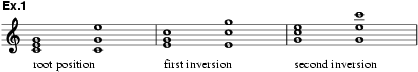
(1) The rearrangement of the notes of a chord built in 3rds so that the lowest note is not the root of the chord. Ex.1 shows the three positions of the C major triad, each in both close and open spacings. If the lowest note of the chord is the 3rd of the triad it is said to be ‘in first inversion’ (see Sixth chord). If its lowest note is the 5th, then it is ‘in second inversion’ (see Six-four chord). The inversion of triads can be extended to 7th chords, where an extra position of the chord – ‘third inversion’, with the 7th as lowest note – is possible (ex.2). Much the same can be applied to 9th chords. In Roman numeral chord notation (see Harmony, §2(ii)), inversions are indicated by arabic numerals or letters: in a C major context, for example, the three chords in ex.1 are either I, I6 and I6-4 or I, Ib and Ic, while ex.2 shows I7, I7b, I7c and I7d.


(2) The complement of an interval with respect to some fixed interval often assumed to be an octave. Within an octave, a 2nd inverts to a 7th, a 3rd to a 6th, a 4th to a 5th and vice versa; within a 10th, a 2nd inverts to a 9th, a 3rd to a an octave, etc. This type of inversion is the basis of Invertible counterpoint, where the functioning of different polyphonic parts as the bass part is dependent on the consonant intervals between them inverting to other consonant intervals.
(3) The mirroring of a succession of notes about a fixed note, usually the first note or interval in the succession of the most easily identified form of inversion because of its association with melodic contour. It is a common feature of contrapuntal music, since it offers new thematic combinations, for whose workability a structurally sound original is usually a necessary and sufficient condition. For some writers the discipline of invertible counterpoint also includes counterpoint based on thematic inversion. This type of inversion has been extended by the theory of Twelve-note composition to include the inversion of the 12 pitch classes making up a set about a fixed pitch class. Thus the set used at the beginning of each movement of Schoenberg’s Suite op.25, shown in ex.4a, yields the set given in ex.4b when inverted about its first note.
WILLIAM DRABKIN